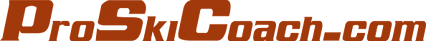Announcement
We've Moved
The forum here @ PSC has been fun, but we're going to transplant it in much more fertile pastures.If you already have a PSC account, use your email address to Request A New Password to get started @wetJacket
#1 Wed, Sep 30, 2009 8:32 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Schnitz: "Are you doing this?"
great stuff!!!
From The Schnitz:
ABOUT THE PHOTO ABOVE
A is where the right entrance gate and #1 line up.
B is where the left entrance gate and #1 line up.
C is where Rossi started displacing water to the outside or prior to #1 being in the middle of the gates. Notice how the boat is still about a half a boat length before the gates - D.
If you back both Rossi and the boat up about 30' (which appears to be the distance back from where he is in the photo) to C, the boat will be about two boat lengths out. This is how early many of the pros begin their movement toward the gates. This applies more and more as the line gets shorter and the speeds increase. I am trying to begin my motion toward the gates when #1 is in the middle at every line length. (Hint; you need your hips forward and your elbows touching your vest or you will miss the gates)
-SCHNITZ!
Offline
#2 Wed, Sep 30, 2009 10:35 PM
- Deke
- Karma: 3
- Slalom Mentor
- Skis At: 15/34
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
Wade,
It sure is "great stuff". I'd like to hear more about though. It would seem that the one handed gate has a lot to do with how early he "displaces water to the outside." A two handed gate would have you flat and unable to begin moving until later. One handed, you are moving out to apex and more able to move in earlier. Am I getting this???
Deke
#3 Wed, Sep 30, 2009 10:47 PM
- Thomas Wayne
- Karma: 5
- Slalom Mentor
Re: Schnitz: "Are you doing this?"
I think it's particularly difficult to apply as much analysis to a single photo as Schnitz seems to often do, and I also think he tends to color his analysis to fit his current "theory du jour". Note that I believe Schnitz is VERY knowledgeable about the physics of skiing, and I don't in any way mean this as a personal attack, but rather to say that there is certainly more than one way to interpret the above photo.
For example, I disagree that "C" is 30 feet from Rossi's present position; I put it at more like 15' - 20', and if you allow that Chris is about 6' tall and imagine laying him down [backwards] onto his ski path I think you'll agree that the distance to "C" is nowhere near FIVE of his lengths.
Also, "displacing water to the outside" is NOT, in and of itself, an absolute indication of a movement back toward the gate. Water begins being displaced to the outside at the very instant an edge change occurs [following the wake crossing] and we all know the skier is still moving outward - AWAY from the wakes [gate] - for quite some time after his ski begins this displacement.
Finally, and most importantly, understanding how Rossi's gate works MUST be applied to this photo. Given his incredible efficiency in initiating his COM movement outward and the fact that he has a very smooth one-handed gate transition, I would argue that he's only actually begun his movement back toward the gate at right about where the #1/B line intersects his ski's path. When the ski is on it's "turning" edge - but still moving OUTWARD - it leaves a distinctive curl to the boat-side edge of the ski track that is unmistakable - note the "white water" appearance right up to the #1/B line. Therefore, not coincidentally, the track left in the water clearly indicates that his ski is continuing to move outward right up to that point.
Frankly, my take on this photo is to conclude that his actual turn in began right at the point where the 1-ball and the left-hand gate lined up, and I think the inherent hydrodynamics involved pretty much proves it.
TW
Last edited by Thomas Wayne (Thu, Oct 1, 2009 4:36 PM)
#4 Thu, Oct 1, 2009 12:06 AM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
Thomas Wayne wrote:
most importantly, understanding how Rossi's gate works MUST be applied to this photo. Given his incredible efficiency in initiating his COM movement outward and the fact that he has a very smooth one-handed gate transition, I would argue that he's only actually begun his movement back toward the gate at right about where the #1/B line intersects his ski's path. When the ski is on it's "turning" edge - but still moving OUTWARD - it leaves a distinctive curl to the boat-side edge of the ski track that is unmistakable - note the "white water" appearance right up to the #1/B line. Therefore, not coincidentally, the track left in the water clearly indicates that his ski is continuing to move outward right up to that point.
TW
Absolutely Correct!
Offline
#5 Thu, Oct 1, 2009 1:39 PM
- jbski
- Karma: 3
- Regular
Re: Schnitz: "Are you doing this?"
TWayne - don't you mean he started his turn in where the left-hand gate and #1 line up, as in where the B line and his path intersect?
Jim
#6 Thu, Oct 1, 2009 1:44 PM
- StevenHaines
- Karma: 11
- Slalom Mentor
- Skis At: Canyon Lake, Ca.
- Foot Forward: right
Re: Schnitz: "Are you doing this?"
Wade, I'm trying to understand this comment.
I am trying to begin my motion toward the gates when #1 is in the middle at every line length. (Hint; you need your hips forward and your elbows touching your vest or you will miss the gates)
What do you mean when you say #1 is in the middle?
And hips forward elbows touching vest, where should this occur?
I've been trying to figure out my gates this year. I'm having trouble with my -38 start (or my issues really surface here) I try to get up on the boat and make an easy turn in for the gates, but find myself with little or no tension on the rope to the point of almost having slack. I've noticed that at the point of reach/turn in, I've been reaching forward and allowing my backside to fall behind and not getting a good counter (like you would in the turn) I've been working on this (countering an pushing my R hip forward) and it helps but still think I have a timing issue. My approach has been, pull out at the 55's with the same intensity, reach/counter when I feel tension on the rope and then try to turn back in towards the wakes with little or no intensity. It seems that the less I try (intensity wise) I have better results. I know, I need a lesson BIG TIME!!!
Thanks Man!
Last edited by Steven Haines (Thu, Oct 1, 2009 1:48 PM)
#7 Thu, Oct 1, 2009 2:02 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
Steve,
I think the diagram is interesting to think about. Above are Schnitz's comments. Maybe he will swing by and give us some more insight soon.
Offline
#8 Thu, Oct 1, 2009 2:28 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
My major point on this is that when you take the overhead view, this looks pretty different. Here is a to-scale diagram. 
(buoys enlarged from scale for reading purposes)
Offline
#9 Thu, Oct 1, 2009 4:00 PM
- StevenHaines
- Karma: 11
- Slalom Mentor
- Skis At: Canyon Lake, Ca.
- Foot Forward: right
Re: Schnitz: "Are you doing this?"
I like the idea of a visual cue, but the reality of it is, I have a tough time getting a visual of 2,4,6 during my pull out! The only time that I get a visual is when I turn in and assess my angle like in view "C". Everything else that I do is based on feel. I "try" not to turn in until the rope get's taken from me. After thinking about it, that might just be my problem at -38. I might be getting too excited and start the turn in too soon (prior to the apex) Paths A & B don't seem to be condusive to achieving skier path C. I like the theory of coordinates when you relate that to line load, but if I'm supposed to be following path A or B (somewhat) it would seem that it would put me on a down course line which would cause my brain to say "Hurry" which is not what I really want to do! As I said before, if I reduce my efforts (try not to try) I have a much better effect and buoy count.
#10 Thu, Oct 1, 2009 4:27 PM
- Schnitz
- Karma: 0
- Slalom Mentor
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
I disagree with the illustration Wade posted above on the "theoretical best line" through the gate, as the skier's speed would be off the charts. As angle increases, speed increases, it's a mathematical equation (distance travelled x time = speed). There comes a point of diminishing return on both sides; too much speed is no good nor is too little. What Chris Rossi is doing is skiing a line that gives him the correct amount of speed for what he is trying to accomplish. He could easily get more angle or less but he has chosen a path that produces the results he is comfortable with! He does this all the way down the course. Being that maintainable cross course angle and speed are directly related, load must also be. Being that load is the enemy, we must do everything within our power to ski as smoothly as possible with the most efficient angle, not the most.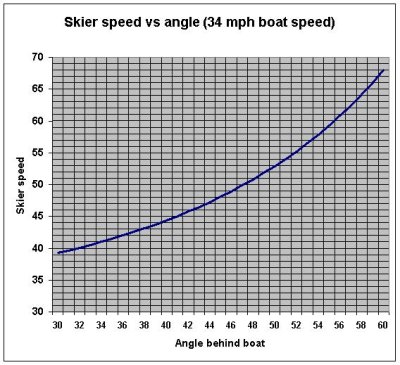
#11 Thu, Oct 1, 2009 4:40 PM
- Thomas Wayne
- Karma: 5
- Slalom Mentor
Re: Schnitz: "Are you doing this?"
WadeWilliams wrote:
My major point on this is that when you take the overhead view, this looks pretty different. Here is a to-scale diagram.
(buoys enlarged from scale for reading purposes)
Unless I'm looking at a different photo than you are, point "C" falls BETWEEN point "A" and point "B", which is not how you've illustrated it...
TW
#12 Thu, Oct 1, 2009 4:41 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
Thomas Wayne wrote:
Unless I'm looking at a different photo than you are, point "C" falls BETWEEN point "A" and point "B", which is not how you've illustrated it...
Correct again! Schnitz's lines are not level with the water's surface. Mine are.
Offline
#13 Thu, Oct 1, 2009 4:45 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
Schnitz wrote:
I disagree with the illustration Wade posted above on the "theoretical best line" through the gate, as the skier's speed would be off the charts. As angle increases, speed increases, it's a mathematical equation (distance travelled x time = speed). There comes a point of diminishing return on both sides; too much speed is no good nor is too little.
I agree with your disagreement!
By "theoretical" best line I meant just exactly what you've written here. There has to be balance between excessive load and speed and the best line. If our technology allowed us, we could take the theoretical best line, without the load that we currently get by pushing ourselves too close to the theoretical line.
Schnitz also wrote:
What Chris Rossi is doing is skiing a line that gives him the correct amount of speed for what he is trying to accomplish. He could easily get more angle or less but he has chosen a path that produces the results he is comfortable with! He does this all the way down the course. Being that maintainable cross course angle and speed are directly related, load must also be. Being that load is the enemy, we must do everything within our power to ski as smoothly as possible with the most efficient angle, not the most.
The closer you can push yourself to my theoretical best line, the earlier you'll be to one, but Schnitz is correct -- there is a diminishing return. We should strive to ski as close to the theoretical best line as possible, while still minimizing the load from the boat. If you're loading turning in, you're too close to the theoretical line.
Offline
#14 Thu, Oct 1, 2009 5:24 PM
- Deke
- Karma: 3
- Slalom Mentor
- Skis At: 15/34
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
I don't agree with the theoretical "best" line at all. I would call it the theoretical "latest" line. This is going where the square wave discussion went a few years ago. It is the "impossible line" where speed and load would have to equal infinity.
Steven, A and B are not ski lines they are site lines. Just visual cues.
This is a GREAT topic!
Last edited by Deke (Thu, Oct 1, 2009 5:26 PM)
#15 Thu, Oct 1, 2009 5:41 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
TO ski around the most buoys @ the shortest line we need the most amount of speed. Hence, "theoretical" "best" line.
Practically, this line is impossible. Don't try to take it! Our skis dont accellerate fast enough, so we are stuck holding all that load which will not make us ski better.
What Schnitz is pointing out -- i think -- is just that Rossi is choosing an Obtainable line, which may not put him on the "theoretical best" line -- which is impossible to ride in the first place. Being on an obtainable line, with minimal load from the boat, will put you in a GOOD ENOUGH position into 1 every time.
Offline
#16 Thu, Oct 1, 2009 6:02 PM
- Deke
- Karma: 3
- Slalom Mentor
- Skis At: 15/34
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
You might argue that the theoretical best line would be straight from one buoy to the next. This would be minimum load, minimum distance travelled, and minimum speed. In theory, all of those parameters would be CONSTANT. The only acelleration would be angular. OTOH in the square wave scenario all of those parameters would be INFINITE. We have a better chance of developing technology and technique to be CONSTANT than we do of being INFINITE!
#17 Thu, Oct 1, 2009 6:08 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
Deke wrote:
We have a better chance of developing technology and technique to be CONSTANT than we do of being INFINITE!
Absolutely. The "Square Wave" is a great solution to run a lot of buoys, but in practice it's impossible.
Incase some of you are confused on the square wave here's a quick diagram:
______ _____
| |
| |
|_____|
Obviously this line is impossible to achieve but it would be the best way to run the most number of buoys... Theoretically, ofcourse.
The Z Wave, direct from buoy to buoy is also impossible (and not theoretically the best) because it doesnt give you any room for error. Our skis will never turn like this -- they are busy sliding in the water the whole time.
\ /\
\ / \
\ / \
\ / \
\/ \
Offline
#18 Thu, Oct 1, 2009 6:09 PM
- Thomas Wayne
- Karma: 5
- Slalom Mentor
Re: Schnitz: "Are you doing this?"
Below is an illustration created from a CAD drawing of the course. It's to exact scale, except for the buoy diameters (per Wade's example). The active elements shown are:
1) Wade's "theoretical best-gate [optimum] line" (broken purple line)
2) Sight lines drawn through the 1-ball and each gate buoy (broken light blue lines), with their corresponding points (“A” & “B”) on the 2-4-6 line
3) "C" is placed where I interpret it's position from Schnitz's photo and from his description: "C is where Rossi started displacing water to the outside or prior to #1 being in the middle of the gates" (never presuming to know what Steve is thinking, I could be wrong about this interpretation)
4) A skier's theoretical path incorporating a wake crossing of 35 degrees and a turn-in radius of 15 feet (solid dark blue line)
Notes:
a) the "theoretical best-gate line" presumes the ski brushing both gate balls, achieving maximum possible angle; in reality this is impossible, but if it was possible the angle would even greater than shown because [in the real world] the gate balls separate by several extra inches due to the boat wake.
b) the sight lines through the 1-ball can only be approximate, since the skier's viewpoint is very dependent on his body position - that is to say, head tipped back or tilted sideways a few inches will greatly change how he views this buoy alignment.
c) a wake-crossing angle of 35 degrees was determined by analyzing still shots captured from overhead video of skilled -35' skiers; obviously different skiing style will vary this angle to some degree (pun intended).
d) a turn radius of 15 feet was suggested in articles written by Steve Schnitz during his "skiing by coordinates" days; again, different skiers will undoubtedly produce different radii.
Of course, the reality of skiing cannot be expressed as easily as such CAD drawings would suggest. In the real world every turn (including turning in for the gates) has an ever-changing radius that cannot be expressed in terms of a simple arc, and there are no long straight lines in the skier's path (excluding a glide following pullout).
I believe that the typical skier path is much better described as one continuous right turn blending seamlessly into a continuous left turn blending into a continuous right turn... and so on. For this reason, the angle of crossing can only be an approximation (at best) derived from the ski path during the brief time it is passing directly behind the boat - this is the closest we can come to estimating that angle, since it’s the only time the path is even close to being a straight line (again, excluding a pre-course prolonged glide).
TW
Last edited by Thomas Wayne (Wed, Oct 7, 2009 3:34 AM)
#19 Thu, Oct 1, 2009 6:15 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
More great stuff.
I think that the closer C can be to the theoretical line, while maintaining minimal line load all the way through the turn in, the better.
Offline
#20 Thu, Oct 1, 2009 6:57 PM
- Schnitz
- Karma: 0
- Slalom Mentor
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
Again, I disagree with Wade but agree at the same time. You see, I want to ski the perfect line, which is both the fastest, and slowest line combined. Any deviation from the perfect line is inefficient. Therefore, any speed faster or slower than the perfect line is detrimental to performance! If 90 degrees were obtainable from one side of the course to the other, the skier would need to be going faster than the speed of light. This would be the fastest line. This would not be beneficial nor would skiing at the next buoy. Somewhere in between lies the perfect line. Think of the slalom course as a target. For the easy speeds and line lengths, you just need to hit the target somewhere. As the speeds and line lengths become harder, the skier needs more accuracy until finally, a bulls eye is necessary every time!
#21 Thu, Oct 1, 2009 7:06 PM
- WadeWilliams
- Karma: 15
- Pro Skier

- From: Lynn, MA
- Registered: Tue, May 15, 2007
- Posts: 1087
- Skis At: Not short enough
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
Agree to disagree. When in Rome.
Offline
#22 Thu, Oct 1, 2009 9:47 PM
- jbski
- Karma: 3
- Regular
Re: Schnitz: "Are you doing this?"
Chuck Norris could ski the square wave. Believe it.
#23 Fri, Oct 2, 2009 12:05 AM
- JAS
- Karma: 0
- Slasher
Re: Schnitz: "Are you doing this?"
Nice discussion but not sure what you can actually take from photo (taken with telephoto lens from grassy knoll). One example of discrepancy is distance on line "A" from gate to ball one, compared to distance on like"B" from gate to ball one. Certainly not the same as Wade's to scale diagram. Warren commission will have plenty to talk about.
#24 Fri, Oct 2, 2009 1:22 AM
- Thomas Wayne
- Karma: 5
- Slalom Mentor
Re: Schnitz: "Are you doing this?"
JAS wrote:
Nice discussion but not sure what you can actually take from photo (taken with telephoto lens from grassy knoll). One example of discrepancy is distance on line "A" from gate to ball one, compared to distance on like"B" from gate to ball one. Certainly not the same as Wade's to scale diagram. Warren commission will have plenty to talk about.
Assume "A" to be the point where the line running through the centers of the 1-ball and the right-hand gate ball intersects the 2-4-6 line, and assume "B" to be the point where the line running through the centers of the 1-ball and the left-hand gate ball intersects the 2-4-6 line. Further assume a course set to exact nominal dimensions.
The distance between A and B in the real world is almost exactly 39 feet.
TW
Last edited by Thomas Wayne (Wed, Oct 7, 2009 3:36 AM)
#25 Fri, Oct 2, 2009 10:00 PM
- ChrisRossi
- Karma: 7
- Pro Skier
- Foot Forward: Right
Re: Schnitz: "Are you doing this?"
My only thought to add to this discussion is that the shorter the rope gets, the later the skiers apex must come. The time my ski can spend outside the buoy line at 41 and 43 is extremely minimal. If I break the buoy line too early, I will end up narrow of the buoy by the time I get to it.